Investment Decisions Notes & Practice Questions CMA

NPV is the result of calculations that find the current value of a future stream of payments using the proper discount rate. In general, projects with a positive NPV are worth undertaking, while those with a negative NPV are not. The first step involved in the calculation of NPV is the estimation of net cash flows from the project over its life. In this example, the NPV is $8,805, which means the project is expected to generate a positive return of $6,805. In this example, the NPV is $8,250, meaning the project is expected to generate a positive return of $6,250. Knowing how to calculate and interpret the NPV of a project can help you make better business decisions and ensure that you’re investing in projects that are likely to yield positive returns.

What is the NPV Formula?

Understanding the role of cost of capital is critical for making informed strategic and financial decisions. No matter how the discount rate is determined, a negative NPV shows that the expected rate of return will fall short of it, meaning that the project will not create value. Net present value (NPV) method (also known as discounted cash flow method) is a popular capital budgeting free invoice templates technique that takes into account the time value of money. The NPV formula is a way of calculating the Net Present Value (NPV) of a series of cash flows based on a specified discount rate. The NPV formula can be very useful for financial analysis and financial modeling when determining the value of an investment (a company, a project, a cost-saving initiative, etc.).

More Helpful Resources

However, what if an investor could choose to receive $100 today or $105 in one year? The 5% rate of return might be worthwhile if comparable investments of equal risk offered less over the same period. It accounts for the fact that, as long as interest rates are positive, a dollar today is worth more than a dollar in the future. Once we have the total present value of all project cash flows, we subtract the initial investment on the project from the total present value of inflows to arrive at net present value.

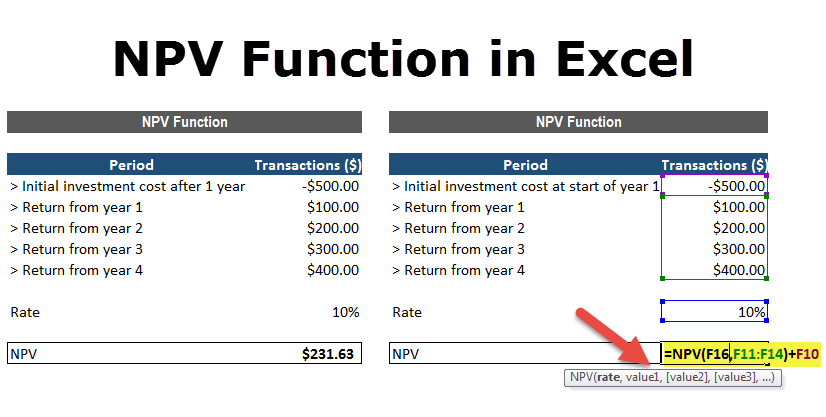
Net present value (NPV) method with uneven cash flow

Therefore, when evaluating investment opportunities, a higher NPV is a favorable indicator, aligning to maximize profitability and create long-term value. One drawback of this method is that it fails to account for the time value of money. For this reason, payback periods calculated for longer-term investments have a greater potential for inaccuracy. Assume the monthly cash flows are earned at the end of the month, with the first payment arriving exactly one month after the equipment has been purchased. This is a future payment, so it needs to be adjusted for the time value of money. An investor can perform this calculation easily with a spreadsheet or calculator.

Comparing Investment Opportunities

• Because the cash inflows and outflows occur in different time periods, they cannot be directly compared to each other.
• This financial model will include all revenues, expenses, capital costs, and details of the business.
• Because the equipment is paid for upfront, this is the first cash flow included in the calculation.
• This assumption might not always be appropriate due to changing economic conditions.

The cost of capital helps companies evaluate potential projects and investments by providing a minimum acceptable return threshold. For example, say Project A requires initial investment of $4 million to generate NPV of $1 million while a competing Project B requires $2 million investment to generate an NPV of $0.8 million. The net cash flows may be even (i.e. equal cash flows in different periods) or uneven (i.e. different cash flows in different periods).

Calculating the NPV of an MBA Program

By paying anything less than $61,000, the investor would earn an internal rate of return that’s greater than 10%. In this example, we are getting a positive net present value of future cash flows, so in this example also we will accept the project. A notable limitation of NPV analysis is that it makes assumptions about future events that may not prove correct. The discount rate value used is a judgment call, while the cost of an investment and its projected returns are necessarily estimates.

Although this is a great tool to use when making investment decisions, it’s not always accurate. Since the equation depends on so many estimates and assumptions, it is difficult to be completely accurate. Going back to our example, Bob has no idea that the interest rate will stay at 10 percent for the next 10 years. He also doesn’t know for sure that he will be able to generate $20,000 of additional revenue from this piece of equipment year over year. The only thing he knows for sure is the price he has to pay for the machine today. NPV is also applied in the valuation of securities, such as bonds, by calculating the present value of their future cash flows and comparing it to the current market price.

Meanwhile, today’s dollar can be invested in a safe asset like government bonds; investments riskier than Treasuries must offer a higher rate of return. However it’s determined, the discount rate is simply the baseline rate of return that a project must exceed to be worthwhile. The payback period calculates how long it will take for the initial investment to be recovered from the project’s cash inflows. In addition, NPV is more accurate when used to compare projects with different lifetimes or risk profiles. It makes comparing investments with different durations easier, as the present value factor is applied uniformly across all cash flows. To calculate NPV, you must know the initial investment and the expected cash flows.